In this article, we’ve compiled over 220 online courses offered by the 60 best universities in the world for studying math in 2024.

We did so by combining popular university rankings to identify the best institutions, and then using the Class Central database to find all their math online courses.

If you’d like to jump to the course list, click here. If you’d like to know how we built the list, keep reading.

Methodology

Manoel built the list following the same data-driven approach used to build the list of computer science courses from the top CS universities.

First, he identified the leading world university rankings. Since we were specifically interested in math, he looked at their latest rankings of the best universities for studying math (or closest superset). Here are the rankings he ended up using:

• QS: World University Ranking 2024 — Mathematics
• Times Higher Education: World University Ranking 2024 — Physical Sciences
• Shanghai Ranking: Academic Ranking of World Universities 2023 — Mathematics

Then, we crawled and scraped each ranking.

Now that we had some data, we used Jupyter with Python to process it. We combined the three rankings into one by averaging the position of each university in each ranking. Then, we filtered out the universities that didn’t offer online courses, and limited the list to the top 60 institutions.

For the 2024 update, Suparn used the same sources and methodology to find the top 60 universities offering online math courses.

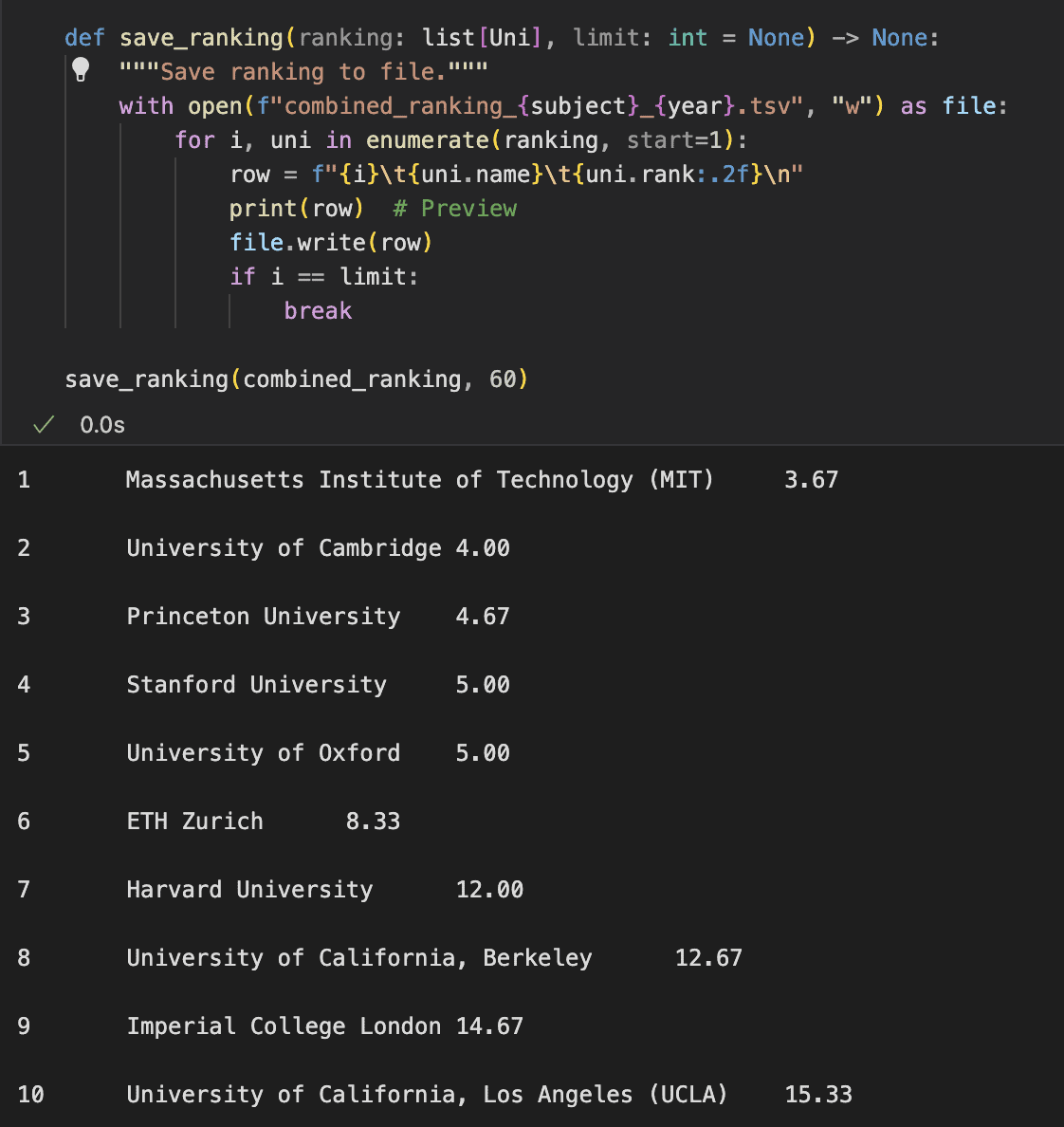
As you can see in the image above, I found that the top three math institutions are:

1. MIT
2. Cambridge
3. Princeton

Finally, we used the Class Central database, with its 250K online courses, to find all the math courses offered by the universities in the ranking.

The end result is a list of more than 220 online courses offered by 60 best universities in the world for studying math in 2024.

While processing the data, I noticed something interesting: 59 of the top 60 universities offer online courses, a lot more than I would have guessed. The world’s top institutions are very prolific creators of online courses.

Stats

• Enrollments range from 11 to over 13 million. There are 8 courses with over 1 million enrollments
• Altogether, the courses in this list have over 50 million enrollments, with an average of over 382 thousand enrollments
• 210 courses are free and 17 are paid
• 211 courses are in English, 8 French, 3 Chinese and 1 each in Spanish, German, Korean, Kazakh, and Russian
• Together, they account for 1,900 reviews at Class Central, with an average of 29 reviews
• Average Rating 4.53 out of 5.0
• 66 courses are beginner level, 45 are intermediate level, and 12 are advanced level.

More Courses

With over 220 courses to pick from, we hope you find something you like. But if these aren’t enough, check out Class Central’s catalog of 250K online courses or our thematic collections:

• 1200+ Free Computer Science Courses from World’s Top Universities
• 25+ Hours of MATLAB Courses with Free Certificate
• 40+ Free Certificates from Wolfram U
• 300 Free Google Certifications
• 10,000+ Free Courses from Tech Giants: Learn from Google, Microsoft, Amazon, and More
• 8000+ OpenCourseWare Courses from Top Institutions.

Subjects

• Linear Algebra (26)
• Differential Equations (6)
• Mathematics (43)
• Vector Calculus (2)
• Foundations of Mathematics (1)
• Statistics & Probability (50)
• Bayesian Statistics (1)
• Calculus (18)
• Precalculus (6)
• Combinatorics (2)
• Linear Regression (4)
• Mathematical Modeling (6)
• Differential Calculus (2)
• Graph Theory (4)
• Multivariable Calculus (3)
• Regression Analysis (2)
• Algebra (4)
• Trigonometry (1)
• Geometry (2)
• Discrete Mathematics (3)
• Linear Programming (1)
• Predictive Modelling (2)
• Integral Calculus (1)
• Chaos Theory (1)
• Numerical Methods (1)
• Single-variable Calculus (1)
• Real Analysis (2)
• Convex Optimization (16)
• Functional Analysis (1)
• Applied Mathematics (2)
• Statistical Analysis (1)
• Probability (1)
• Group Theory (1)
• Engineering Mathematics (2)
• Finite Fields (1)
• Ordinary Differential Equations (1)
• Taylor Series (1)
• Statistical Methods (1)
• Knot Theory (1)
• Number Theory (2)
• Mathematical Puzzles (1)

Linear Algebra (26)

• Matrix Algebra for Engineers from The Hong Kong University of Science and Technology ★★★★★(749)
• Linear Algebra – Foundations to Frontiers from The University of Texas at Austin ★★★★☆(16)
• Introduction to Linear Models and Matrix Algebra from Harvard University ★★★★☆(12)
• Mathematics for Machine Learning: Linear Algebra from Imperial College London ★★★☆☆(9)
• Linear Algebra from Massachusetts Institute of Technology ★★★★★(3)
• Matrix Methods in Data Analysis, Signal Processing, and Machine Learning from Massachusetts Institute of Technology ★★★★★(3)
• Algèbre Linéaire (Partie 1) from École Polytechnique Fédérale de Lausanne ★★★★★(2)
• Algèbre Linéaire (Partie 2) from École Polytechnique Fédérale de Lausanne ★★★★★(1)
• Algèbre Linéaire (Partie 3) from École Polytechnique Fédérale de Lausanne ★★★★★(1)
• Linear Algebra III: Determinants and Eigenvalues from Georgia Institute of Technology ★★★★★(1)
• Linear Algebra IV: Orthogonality & Symmetric Matrices and the SVD from Georgia Institute of Technology ★★★★★(1)
• MIT A 2020 Vision of Linear Algebra, Spring 2020 from Massachusetts Institute of Technology ★★★★★(1)
• Applications of Linear Algebra from Georgia Institute of Technology ★★★★★(1)
• Linear Algebra: Linear Systems and Matrix Equations from Johns Hopkins University
• Linear Algebra: Matrix Algebra, Determinants, & Eigenvectors from Johns Hopkins University
• Linear Algebra I: Linear Equations from Georgia Institute of Technology
• Linear Algebra II: Matrix Algebra from Georgia Institute of Technology
• Linear Algebra: Orthogonality and Diagonalization from Johns Hopkins University
• Advanced Linear Algebra: Foundations to Frontiers from The University of Texas at Austin
• MIT 2.087 Engineering Mathematics: Linear Algebra and ODEs, Fall 2014 from Massachusetts Institute of Technology
• Linear Algebra from Elementary to Advanced from Johns Hopkins University
• Introductory Linear Algebra from Georgia Institute of Technology
• Linear Algebra from Massachusetts Institute of Technology
• Matrix Calculus for Machine Learning and Beyond from Massachusetts Institute of Technology
• A Vision of Linear Algebra from Massachusetts Institute of Technology
• Computational Science and Engineering I from Massachusetts Institute of Technology

Differential Equations (6)

• Differential Equations for Engineers from The Hong Kong University of Science and Technology ★★★★★(329)
• Differential Equations from Massachusetts Institute of Technology ★★★★★(2)
• 18.03x Differential Equations from Massachusetts Institute of Technology
• Numerical Solution of Differential Equations: Oxford Mathematics 3rd Year Student Lecture from University of Oxford
• Engineering Math: Differential Equations and Linear Algebra from Massachusetts Institute of Technology
• Differential Equations from Massachusetts Institute of Technology

Mathematics (43)

• Fibonacci Numbers and the Golden Ratio from The Hong Kong University of Science and Technology ★★★★★(252)
• How to Learn Math: For Students from Stanford University ★★★★☆(17)
• Convex Optimization from Stanford University ★★★★★(8)
• Mathematics for Engineers: The Capstone Course from The Hong Kong University of Science and Technology ★★★★★(5)
• A-level Mathematics for Year 12 – Course 1: Algebraic Methods, Graphs and Applied Mathematics Methods from Imperial College London ★★★★★(2)
• Mathematical Thinking in Computer Science from University of California, San Diego ★★★★★(2)
• Mathematics for Engineers from The Hong Kong University of Science and Technology ★★★★★(1)
• Essential Math for AI from Columbia University
• Cómo Aprender Matemáticas – Para Estudiantes from Stanford University
• A-level Further Mathematics for Year 12 – Course 1: Complex Numbers, Matrices, Roots of Polynomial Equations and Vectors from Imperial College London
• Delivery Problem from University of California, San Diego
• A-level Further Mathematics for Year 13 – Course 1: Differential Equations, Further Integration, Curve Sketching, Complex Numbers, the Vector Product and Further Matrices from Imperial College London
• Further Mathematics Year 13 course 2: Applications of Differential Equations, Momentum, Work, Energy & Power, The Poisson Distribution, The Central Limit Theorem, Chi Squared Tests, Type I and II Errors from Imperial College London
• Introduction to optimization on smooth manifolds: first order methods from École Polytechnique Fédérale de Lausanne
• Introduction to Complexity Science from Nanyang Technological University
• Mathematics of Waves: Visualized with Neural Networks from Purdue University
• Nonlinear Dynamics and Chaos from Cornell University
• MIT RES.LL-005 Mathematics of Big Data and Machine Learning, IAP 2020 from Massachusetts Institute of Technology
• Engineering Mathematics (UW ME564 and ME565) from University of Washington
• 离散数学 from Shanghai Jiao Tong University
• How Learning Ten Equations Can Improve Your Life – David Sumpter from University of Oxford
• Analyse I (partie 4) : Limite d’une fonction, fonctions continues from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 5) : Fonctions continues et fonctions dérivables, la fonction dérivée from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 6) : Etudes des fonctions, développements limités from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 1) : Prélude, notions de base, les nombres réels from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 2) : Introduction aux nombres complexes from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 3) : Suites de nombres réels I et II from École Polytechnique Fédérale de Lausanne
• Analyse I (partie 7) : Intégrales indéfinies et définies, intégration (chapitres choisis) from École Polytechnique Fédérale de Lausanne
• Wrinkling: Oxford Mathematics Research Seminar from University of Oxford
• Stanford Lecture: Don Knuth – “Pi and The Art of Computer Programming” (2019) from Stanford University
• Cascading Principles – Conrad Shawcross, Martin Bridson and James Sparks with Fatos Ustek from University of Oxford
• Why Does Rudolph Have A Shiny Nose? – Chris Budd from University of Oxford
• Bach and the Cosmos from University of Oxford
• The Mathematics of Visual Illusions – Ian Stewart from University of Oxford
• Prime Time – James Maynard from University of Oxford
• Théorie des Groupes (partie 1) – Une introduction à la théorie des catégories from École Polytechnique Fédérale de Lausanne
• Political Geometry: The Mathematics of Redistricting | Moon Duchin || Radcliffe Institute from Harvard University
• Introduction to Metric Spaces from Massachusetts Institute of Technology
• Productive generalization – Timothy Gowers from University of Oxford
• The Num8er My5teries – Marcus du Sautoy from University of Oxford
• Euler’s pioneering equation from University of Oxford
• The Travelling Santa Problem and Other Seasonal Challenges – Marcus du Sautoy from University of Oxford
• The Potential for AI in Science and Mathematics – Terence Tao from University of Oxford

Vector Calculus (2)

• Vector Calculus for Engineers from The Hong Kong University of Science and Technology ★★★★★(245)
• Calculus through Data & Modelling: Vector Calculus from Johns Hopkins University

Foundations of Mathematics (1)

• Introduction to Mathematical Thinking from Stanford University ★★★★☆(51)

Statistics & Probability (50)

• Intro to Statistics from Stanford University ★★★★☆(39)
• Probability – The Science of Uncertainty and Data from Massachusetts Institute of Technology ★★★★★(34)
• Statistical Learning with R from Stanford University ★★★★☆(28)
• Fundamentals of Statistics from Massachusetts Institute of Technology ★★★★☆(10)
• Fat Chance: Probability from the Ground Up from Harvard University ★★★★☆(6)
• Introduction to Probability and Data with R from Duke University ★★★★☆(6)
• Statistical Inference and Modeling for High-throughput Experiments from Harvard University ★★★★★(4)
• Computational Probability and Inference from Massachusetts Institute of Technology ★★★★★(3)
• Introduction to Statistics from Stanford University ★★★★★(2)
• Statistics: Unlocking the World of Data from University of Edinburgh ★★★★☆(2)
• Introduction to Probability from Harvard University ★★★★★(1)
• Introduction to Statistics & Data Analysis in Public Health from Imperial College London ★★★★★(1)
• Summary Statistics in Public Health from Johns Hopkins University ★★★★★(1)
• Hypothesis Testing in Public Health from Johns Hopkins University ★★★★★(1)
• A Crash Course in Causality: Inferring Causal Effects from Observational Data from University of Pennsylvania ★★★★☆(1)
• Statistics for Applications from Massachusetts Institute of Technology ★★★★★(1)
• Causal Inference from Columbia University
• Causal Inference 2 from Columbia University
• Probability: Basic Concepts & Discrete Random Variables from Purdue University
• Probability: Distribution Models & Continuous Random Variables from Purdue University
• Survival Analysis in R for Public Health from Imperial College London
• Logistic Regression in R for Public Health from Imperial College London
• Statistics for Data Science Essentials from University of Pennsylvania
• Introduction to Probability Management from Stanford University
• Statistics Using Python from University of Wisconsin–Madison
• Probability and Statistics I: A Gentle Introduction to Probability from Georgia Institute of Technology
• Inferential Statistics from Duke University
• Statistics 110: Probability from Harvard University
• Probability and Statistics III: A Gentle Introduction to Statistics from Georgia Institute of Technology
• What are the Chances? Probability and Uncertainty in Statistics from Johns Hopkins University
• Probability and Statistics IV: Confidence Intervals and Hypothesis Tests from Georgia Institute of Technology
• Advanced Statistics for Data Science from Johns Hopkins University
• Probability and Statistics II: Random Variables – Great Expectations to Bell Curves from Georgia Institute of Technology
• Statistics with Python from University of Michigan
• Probabilistic Systems Analysis and Applied Probability from Massachusetts Institute of Technology
• Probabilistic Graphical Models from Stanford University
• Selected Topics on Discrete Choice from École Polytechnique Fédérale de Lausanne
• Probabilistic Systems Analysis and Applied Probability from Massachusetts Institute of Technology
• MIT RES.6-012 Introduction to Probability, Spring 2018 from Massachusetts Institute of Technology
• Bayesian Networks 3 – Maximum Likelihood | Stanford CS221: AI (Autumn 2019) from Stanford University
• Bayesian Networks 2 – Forward-Backward | Stanford CS221: AI (Autumn 2019) from Stanford University
• Bayesian Networks 1 – Inference | Stanford CS221: AI (Autumn 2019) from Stanford University
• Statistics, Confidence Intervals and Hypothesis Tests from Georgia Institute of Technology
• Probability/Random Variables from Georgia Institute of Technology
• Modèles de durée from Université Paris-Saclay
• Stanford Webinar – Data Overload: Making Sense of Statistics in the News, Kristin Sainani from Stanford University
• Inferenzstatistik from Duke University
• R을 사용한 확률 및 데이터 소개 from Duke University
• Brooklyn Quant Experience Lecture Series: Alejandra Quintos Lima from New York University (NYU)
• The Counting Project – Tim Harford from University of Oxford

Bayesian Statistics (1)

• Bayesian Statistics from Duke University ★★★☆☆(12)

Calculus (18)

• Calculus: Single Variable Part 1 – Functions from University of Pennsylvania ★★★★★(8)
• Calculus: Single Variable Part 2 – Differentiation from University of Pennsylvania ★★★★★(5)
• Calculus: Single Variable Part 3 – Integration from University of Pennsylvania ★★★★☆(4)
• Calculus: Single Variable Part 4 – Applications from University of Pennsylvania ★★★★★(3)
• Calculus Applied! from Harvard University ★★★★★(2)
• Combinatorial Mathematics | 组合数学 from Tsinghua University ★★★★☆(2)
• Single Variable Calculus from Massachusetts Institute of Technology ★★★★★(2)
• Calculus through Data & Modeling: Differentiation Rules from Johns Hopkins University ★★★★☆(1)
• Introductory Calculus: Oxford Mathematics 1st Year Student Lecture from University of Oxford ★★★★★(1)
• Single Variable Calculus from University of Pennsylvania
• Applied Calculus with Python from Johns Hopkins University
• Calculus through Data & Modeling: Precalculus Review from Johns Hopkins University
• Calculus through Data & Modeling: Limits & Derivatives from Johns Hopkins University
• A-Level Further Mathematics for Year 12 – Course 2: 3 x 3 Matrices, Mathematical Induction, Calculus Methods and Applications, Maclaurin Series, Complex Numbers and Polar Coordinates from Imperial College London
• Calculus through Data & Modelling: Series and Integration from Johns Hopkins University
• Calculus through Data & Modeling: Applying Differentiation from Johns Hopkins University
• Calculus through Data & Modelling: Integration Applications from Johns Hopkins University
• Calculus through Data & Modelling: Techniques of Integration from Johns Hopkins University

Precalculus (6)

• Discovery Precalculus: A Creative and Connected Approach from The University of Texas at Austin ★★★★★(3)
• Precalculus from Modern States ★★★★★(1)
• Precalculus: Relations and Functions from Johns Hopkins University
• Precalculus: Mathematical Modeling from Johns Hopkins University
• Precalculus: Periodic Functions from Johns Hopkins University
• Precalculus through Data and Modelling from Johns Hopkins University

Combinatorics (2)

• Combinatorics and Probability from Moscow Institute of Physics and Technology ★★★★☆(3)
• Analytic Combinatorics from Princeton University ★★★★☆(3)

Linear Regression (4)

• Data Science: Linear Regression from Harvard University ★★☆☆☆(3)
• Linear Regression and Modeling from Duke University ★★★★☆(2)
• Linear Regression in R for Public Health from Imperial College London ★★★★★(1)
• Linear Regression Modeling for Health Data from University of Michigan

Mathematical Modeling (6)

• Modelling Ice Sheets: Oxford Mathematics Research Seminar from University of Oxford ★★★★★(3)
• Infectious Disease Modeling in Practice from Johns Hopkins University
• Scaling the Maths of Life from University of Oxford
• How do mathematicians model infectious disease outbreaks? from University of Oxford
• Mathematical Modelling in Biology: Neuronal Signalling – Oxford Mathematics 2nd Yr Student Lecture from University of Oxford
• Mathematical Modelling in Biology: Enzyme Kinetics & Perturbation Theory – 2nd Year Student Lecture from University of Oxford

Differential Calculus (2)

• Engineering Calculus and Differential Equations from The University of Hong Kong ★★★★★(2)
• Differential Calculus through Data and Modeling from Johns Hopkins University

Graph Theory (4)

• Introduction to Graph Theory from University of California, San Diego ★★★★★(2)
• Graph Theory and Additive Combinatorics from Massachusetts Institute of Technology
• Graph Theory and Additive Combinatorics from Massachusetts Institute of Technology
• Stanford Lecture: Don Knuth—”Hamiltonian Paths in Antiquity” (2016) (360 Degrees) from Stanford University

Multivariable Calculus (3)

• Multivariable Calculus from Massachusetts Institute of Technology ★★★★★(2)
• Multivariable Calculus from Massachusetts Institute of Technology
• Multidimensional Analysis & Geometry: introduction to the derivative in higher dimensions, lecture 1 from University of Oxford

Regression Analysis (2)

• Simple Regression Analysis in Public Health from Johns Hopkins University ★★★★★(1)
• Sarah Bana: Using Language Models to Understand Wage Premia from Stanford University

Algebra (4)

• Algebra: Elementary to Advanced – Functions & Applications from Johns Hopkins University ★★★★☆(1)
• Algebra: Elementary to Advanced from Johns Hopkins University ★★★★★(1)
• Algebra: Elementary to Advanced – Equations & Inequalities from Johns Hopkins University
• Algebra: Elementary to Advanced – Polynomials and Roots from Johns Hopkins University

Trigonometry (1)

• Cours préparatoire: Fonctions Trigonométriques, Logarithmiques et Exponentielles from École Polytechnique Fédérale de Lausanne ★★★★☆(1)

Geometry (2)

• The Seduction of Curves: The Lines of Beauty That Connect Mathematics, Art and The Nude from University of Oxford ★★★★★(1)
• Geometry – Scalar Triple Product: Oxford Mathematics 1st Year Student Lecture from University of Oxford

Discrete Mathematics (3)

• Discrete Mathematics from Shanghai Jiao Tong University
• Introduction to Discrete Mathematics for Computer Science from University of California, San Diego
• 离散数学概论 Discrete Mathematics Generality from Peking University

Linear Programming (1)

• Optimization: principles and algorithms – Linear optimization from École Polytechnique Fédérale de Lausanne

Predictive Modelling (2)

• Meaningful Predictive Modeling from University of California, San Diego
• Performative Modelling from National University of Singapore

Integral Calculus (1)

• Integral Calculus through Data and Modeling from Johns Hopkins University

Chaos Theory (1)

• The Butterfly Effect – What Does It Really Signify? from University of Oxford

Numerical Methods (1)

• Numerical Methods Applied to Chemical Engineering from Massachusetts Institute of Technology

Single-variable Calculus (1)

• Single Variable Calculus from Massachusetts Institute of Technology

Real Analysis (2)

• Real Analysis from Massachusetts Institute of Technology
• The Banach contraction mapping theorem – Oxford Mathematics 1st Year Student Lecture from University of Oxford

Convex Optimization (16)

• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 1 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 3 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 2 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 5 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 4 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 6 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 8 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 7 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 10 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 9 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 12 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 11 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 13 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 17 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 16 from Stanford University
• Stanford EE364A Convex Optimization I Stephen Boyd I 2023 I Lecture 15 from Stanford University

Functional Analysis (1)

• Introduction to Functional Analysis from Massachusetts Institute of Technology

Applied Mathematics (2)

• Why there are no three-headed monsters from University of Oxford
• From Ronald Ross to ChatGPT: the birth and strange life of the random walk – Jordan Ellenberg from University of Oxford

Statistical Analysis (1)

• Stanford Webinar: How to Be a Statistical Detective from Stanford University

Probability (1)

• Stanford CS109 I Future of Probability I 2022 I Lecture 28 from Stanford University

Group Theory (1)

• Théorie des Groupes (partie 2) – Quotients de groupe from École Polytechnique Fédérale de Lausanne

Engineering Mathematics (2)

• Инженерлерге арналған математика from The Hong Kong University of Science and Technology
• Математика для инженеров from The Hong Kong University of Science and Technology

Finite Fields (1)

• Principles of Digital Communication II from Massachusetts Institute of Technology

Ordinary Differential Equations (1)

• Mathematical Methods for Engineers II from Massachusetts Institute of Technology

Taylor Series (1)

• 16. The Taylor Series and Other Mathematical Concepts from Yale University

Statistical Methods (1)

• Social Sequence Analysis: An Overview from The University of Chicago

Knot Theory (1)

• Knotty Problems – Marc Lackenby from University of Oxford

Number Theory (2)

• Numbers are Serious but they are also Fun – Michael Atiyah from University of Oxford
• Linear Diophantine equations and the extended Euclidean algorithm – 1st year student lecture from University of Oxford

Mathematical Puzzles (1)

• Can Yule solve my problems – Alex Bellos from University of Oxford